Hydrodynamic lubrication theory

Dr. Dmitri Kopeliovich

A theoretical analysis of hydrodynamic lubrication was carried out by Osborne Reynolds.
The equations resulted from the analysis has served a basis for designing hydrodynamically lubricated bearings.

The following assumptions were made by Reynolds in the analysis:

• The lubricating fluid is Newtonian - the flow is laminar and the shear stress between the flow layers is proportional to the velocity gradient in the direction perpendicular to the flow (Newton’s law of viscosity):
  

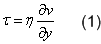
Where:
η – dynamic viscosity of oil,
v – linear velocity of the laminar layer,
y - the axis perpendicular to the flow direction.

• The inertia forces resulted from from the accelerated movement of the flowing lubricant are neglected.
• The lubricating fluid is incompressible.
• The pressure of the fluid p is constant in the direction perpendicular to the laminar flow: dp/dy=0 (assumption of thin lubrication film).
• The viscosity of the fluid is constant throughout the lubrication film.

Consider the equilibrium of a unit volume in the lubricant film.



The pressure forces act on the right and the left faces of the unit volume.
The shear forces resulting from the relative motion of the laminar layers act along the upper and the lower faces of the unit volume.
Assuming that there is no flow in z direction the equation of the equilibrium of the forces in the direction of the flow is as follows:

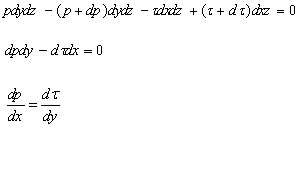
Substitution of τ from Newton’s law of viscosity (1) results in:

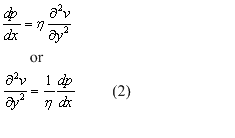
The velocity function is obtained by integrating the equation (2) with respect to y:


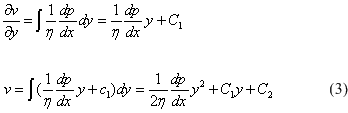
The constants of integration C₁ and C₂ may be determined from the boundary conditions:

v=U when y=0, then C₂=U

v=0 when y=h, then:


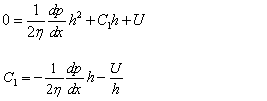
Substituting C₁ and C₂ in (3) we obtain:


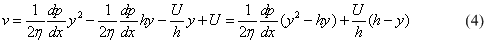
The total flow of the lubricant is:

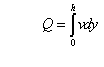
Substituting v from (4) we get:


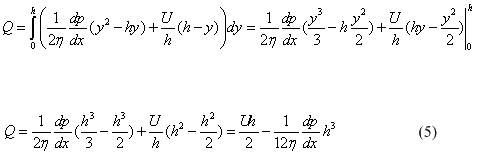
According to the assumption about incompressibility of the lubricant the flow Q does not change in x direction:

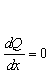
Differentiating the equation (5) with respect x results in:


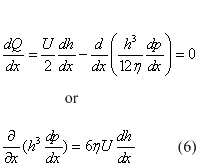
This is Reynolds equation for one dimensional flow.
It can be used with the assumption of no flow in z direction (bearings with infinite length).

If the flow in z direction is taken into account (bearings with side leakage of the lubricating fluid) then the analysis results in Reynolds equation for two dimensional flow:



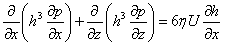
Where:
h – local oil film thickness,
η – dynamic viscosity of oil,
p – local oil film pressure,
U – linear velocity of journal,
x - circumferential direction.
z - longitudinal direction.

Close form solution of Reynolds equation can not be obtained therefore finite elements method is used to solve it.

Analytical solutions of Reynolds equation exist only for certain assumptions:

• Sommerfeld Solution
  

The equation is solved with the assumption that there is no lubricant flow in the axial direction (infinitely long bearing assumption).

• Ocvirk Solution
  

Ocvirk solution for infinitely short bearing assumption neglects circumferential pressure gradients (first term of Reynolds equation).


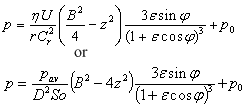
Where:

C_r – radial clearance C_r = (D-D_j)/2,
r – bearing radius,
D_j - journal diameter,
ε - eccentricity ratio ε = e/C_r
e - absolute bearing eccentricity,
B – bearing length,
p₀ - cavitation pressure,
So - Sommerfeld number (see below).

So = F Ψ²/(DBωη)

Where:
F – bearing load,
Ψ – relative bearing clearance Ψ = (D-D_j)/D,
D – bearing diameter,
ω – angular speed of journal.

Sommerfeld and Ocvirk solutions are applicable only in the region of positive pressure.

to top

• Bearings in internal combustion engines
• Engine parameters determining bearing loading
• Lubrication regimes
• Hydrodynamic journal bearing
• Geometrical parameters of engine bearings
• Mechanisms of wear
• Requirements to engine bearing materials
• Engine bearing fatigue test
• Engine bearing materials
• Copper based bearing materials
• Manufacturing bi-metal strips for copper bearings
• Aluminum based bearing materials
• Continuous casting of aluminum based bearing alloys
• Manufacturing bi-metal strips for aluminum bearings
• Lead based engine bearing overlays
• Tin based engine bearing overlays
• Sputter bearing overlays
• Polymer based engine bearing overlays
• Engine bearing failure
• Selection of engine bearing materials
• Effect of low viscosity oils on engine bearings
• pMax Black™ - Strengthened Tri-metal Bearing Materials for High Performance Bearings
• U-Groove™ Modified Design of Oil Groove for High Performance Bearings
• ElliptiX™ Oil Hole of New Design for High Performance Bearings
• EccentriX™ Optimal Eccentricity for High Performance Bearings
• RadiaLock™ - Design of Crush Height for Reliable Press Fit of High Performance Bearings
• Modern Trends In Materials For High Performance Engine Bearings
• Optimization of Clearance Design for High Performance Engine Bearings
• Camshaft Bearings
• Ecological Aspects Of Engine Bearings
• Consistency in Bearing Wall Thickness
• Soft Strength of Racing Bearings
• Effect of oil viscosity on hydrodynamic friction of engine bearings
• Bearing Materials For Race Engines
• Oil Clearance and Engine Bearings
• Polymer Coated Bearings
• Development of Performance Bearings for Race Engines of Modern Design
• Failures of Engine Bearings and their Prevention
• Engine Bearing Housing and How it Affects Engine Bearings
• Polymer Coatings for Race Engine Bearings
• Heavy Duty Engine Bearings - Materials, Geometry, Failures
• Hydrodynamic Bearing – Calculations and Design
• Geometry of Engine Bearings
• Crush Height of Engine Bearings
• Materials for Engine Bearings
• Engine oils

to Metals
to Engine bearings